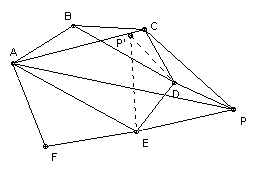

ABCDEF is a convex hexagon with ∠B + ∠D + ∠F = 360o and AB·CD·EF = BC·DE·FA. Show that BC·DF·EA = EF·AC·BD.
Solution

Take P so that AFE and PDE are similar. [So take ∠PDE = ∠AFE and ∠PED = ∠AEF. Note that you need to take the right point - P' fails below.] So EF/ED = EA/EP. Also ∠DEF = ∠DEA + ∠AEF = ∠DEA + ∠PED = ∠PEA (this is where we need P on the opposite side of DE to A). Hence triangles DEF and PEA are similar. So FD/EF = AP/EA (*).
Triangles AFE, PDE similar also gives FA/EF = DP/DE. So AB/BC = (DE/CD) (FA/EF) (given) = (DE/CD) (DP/DE) = PD/DC. But ∠CDE + ∠EDP + ∠PDC = 360o, or ∠D + ∠F + ∠PDC = 360o. We are given that ∠B + ∠D + ∠F = 360o, so ∠PDC = ∠ABC. Hence triangles ABC and PDC are similar. So CB/CD = CA/CP. ∠BCD = ∠ACD + ∠BCA = ∠ACD + ∠DCP = ∠ACP. So triangles BCD and ACP are similar. Hence BC/BD = AC/AP. Multiplying by (*) gives (BC/BD) (FD/EF) = AC/EA, which is the required equality.
![]()
© John Scholes
jscholes@kalva.demon.co.uk
30 Aug 2002